We
the team of subject wise have provided detailed solution of class 10
ncert exercise 3.2 so please read this post completely if you have any
doubt please comment it down also follow our you-tube channel.
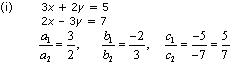
Since , the given pair of equation has only one solution.
, the given pair of equation has only one solution.
Thus, the pair of linear equations is consistent.
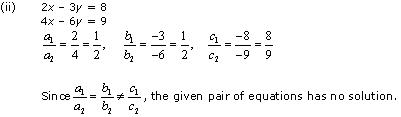
Thus, the pair of linear equations is inconsistent.
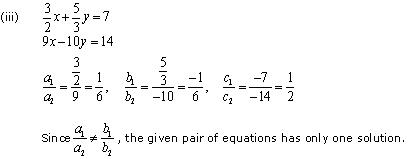
Thus, the pair of linear equations is consistent.
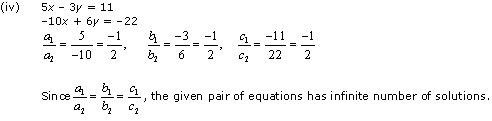
Thus, the pair of linear equations is consistent.
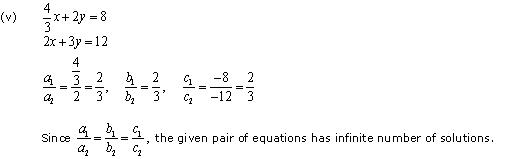
Thus, the pair of linear equations is consistent.
Concept Insight: If a pair of linear equations has one or more than one solution then they are said to be consistent and if they have no solution then they are said to be inconsistent. So, to identify the consistency of a given pair of equations, apply the conditions involving the coefficients of the given pair of equations.
In case, two consistent linear equations are plotted, they will either intersect or overlap each other.
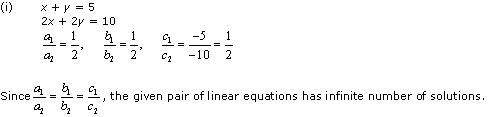
Thus, the pair of linear equations is consistent.
Now, x + y = 5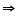 x = 5 - y
x = 5 - y
Three solutions of this equation can be written in a table as follows:
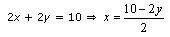
Three solutions of this equation can be written in a table as follows:
Thus, the graphical representation is as follows:
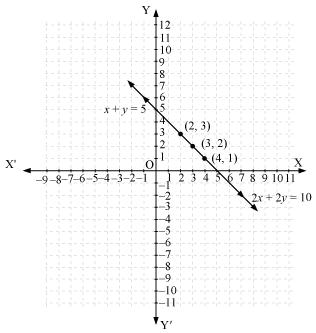
From the graph, it can be observed that the two lines coincide. Thus, the given pair of equations has infinite solutions.
Let x = k, then y = 5 - k. So, the ordered pair (k, 5 - k) , where k is a constant, will be the solution of the given pair of linear equations.
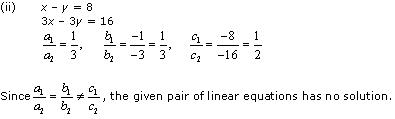
Thus, the pair of linear equations is inconsistent.
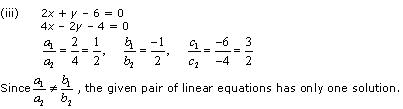
Thus, the pair of linear equations is consistent.
Now, 2x + y - 6 = 0 y = 6 - 2x
y = 6 - 2x
Three solutions of this equation can be written in a table as follows:
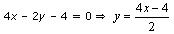
Three solutions of this equation can be written in a table as follows:
Thus, the graphical representation is as follows:
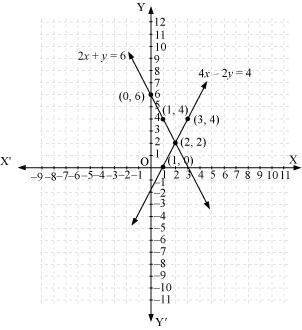
From the graph, it can be observed that the two lines intersect each other at the point (2, 2). Thus, the solution of the given pair of equations is (2, 2).
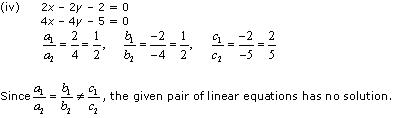
Thus, the pair of linear equations is inconsistent.
Concept insight: If a pair of linear equations has one or more than one solutions then they are said to be consistent and if they have no solution then they are said to be inconsistent. The graph of each equation can be plotted by taking at least three ordered pairs which are the solutions of the equations. The point where both the lines intersect will be the solution of the given pair of equations. Remember two overlapping lines intersect each other at infinitely many points. State the solution explicitly.
 x = y - 1
x = y - 1
Three solutions of this equation can be written in a table as follows:

Three solutions of this equation can be written in a table as follows:
Now, these equations can be drawn on a graph. The triangle formed by the two lines and the x-axis can be shown by the shaded part as:
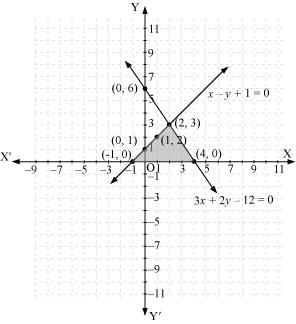
From the graph, it can be observed that the coordinates of the vertices of the triangle so formed are (2, 3), (-1, 0), and (4, 0).
Concept insight: In order to find the coordinates of the vertices of the triangle so formed, find the points where the two lines intersects the x-axis and also where the two lines intersect each other. Note here that the coordinates of the intersection of lines with x-axis is taken and not with y-axis, this is because the question says to find the triangle formed by the two lines and the x-axis.
not forget to suscribe our channel. also follow us on the telegram grouup
Here at Subject Wise Our Focus Is To Provide Best Quality Education To All Students From India And Across The Globe.
------------------------------------------------------------------------------------------------------------
Follow Us On Our Social Media Handles For Behind The Scenes Stuff And Important Updates....
TELEGRAM 👇🏼
https://t.me/joinchat/K40m2h2t4kDvXN1ATNYUqg
Blogger 👇🏼
https://subjectwiseyt.blogspot.com/
-------------------------------------------------------------------------------------------------------------
If This Video Was Helpful Do Leave A Like Since It Encourages Us To Make More Videos For All You Wonderful Students.
Make Sure To Comment Your Views Below.
Lastly Make Sure To Subscribe Our Channel And Press The Bell Icon For All Notification Of Our Latest Videos.
-------------------------------------------------------------------------------------------------------------
For Live Sessions, Chapter wise notes and Important Question Bank make sure you register at
https://subjectwiseyt.blogspot.com/
Get Your Courses On Our Website : For A Distraction Free And Efficient Studies
-------------------------------------------------------------------------------------------------------------
Please help me and tell me all the mistakes committed by me so i can improve it in next video.
Dont Forget to subscribe my channel.
this are the link of previous video-
Basics of Biology Part 1-
https://www.youtube.com/watch?v=R3UzzSq-h2Y
Part2- Electricity Class 10-
https://www.youtube.com/watch?v=Wjsw6GuJnz0&list=PLcMqfTNwNJuQ6ut9yqZwWaHiaPRgJIJ57&index=2
Part 1 Electricity Class 10-
https://youtu.be/JvGpUNF5dhA
Class 10 physics s chand-
https://amzn.to/2ygIG5a
Interesting question video-https://mydomainscan.com/ZsbiLmG
Maths problem-
https://mydomainscan.com/wxSr5VQg
motivation-
https://mydomainscan.com/wxSr5VQg
best books-
https://amzn.to/3fThv1f
https://amzn.to/2LEbPKA
Next video will definetly come within one week
------------------------------------------------------------------------------------------------
SubjectWise - New way to learn
------------------------------------------------------------------------------------------------
At last Thanks for watching Our Videos For any new Requirement please Comment Below.
#Class10Crack #FightagainstCorona #SubjectWise
Chapter 3-Pairs of linear equation in 1 variable Exercise Ex.3.2
here it is solution of question 1
Chapter 3 - Pairs of Linear Equations in Two Variables Exercise Ex. 3.2
Solution 1
(i) Let the number of girls and boys in the class be x and y respectively.
According to the given conditions, we have:
x + y = 10
x - y = 4
x + y = 10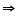 x = 10 - y
x = 10 - y
Three solutions of this equation can be written in a table as follows:
x - y = 4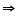 x = 4 + y
x = 4 + y
Three solutions of this equation can be written in a table as follows:
The graphical representation is as follows:
From the graph, it can be observed that the two lines intersect each other at the point (7, 3).
So, x = 7 and y = 3.
Thus, the number of girls and boys in the class are 7 and 3 respectively.
(ii) Let the cost of one pencil and one pen be Rs x and Rs y respectively.
According to the given conditions, we have:
5x + 7y = 50
7x + 5y = 46

Three solutions of this equation can be written in a table as follows:

Three solutions of this equation can be written in a table as follows:
The graphical representation is as follows:
From the graph, it can be observed that the two lines intersect each other at the point (3, 5).
So, x = 3 and y = 5.
Therefore, the cost of one pencil and one pen are Rs 3 and Rs 5 respectively.
Concept insight: Read the question carefully and examine what are the unknowns. Represent the given conditions with the help of equations by taking the unknowns quantities as variables. Also carefully state the variables as whole solution is based on it. On the graph paper, mark the points accurately and neatly using a sharp pencil. Also, take at least three points satisfying the two equations in order to obtain the correct straight line of the equation. Since joining any two points gives a straight line and if one of the points is computed incorrect will give a wrong line and taking third point will give a correct line. The point where the two straight lines will intersect will give the values of the two variables, i.e., the solution of the two linear equations. State the solution point.
According to the given conditions, we have:
x + y = 10
x - y = 4
x + y = 10
Three solutions of this equation can be written in a table as follows:
| x | 5 | 4 | 6 |
| y | 5 | 6 | 4 |
x - y = 4
Three solutions of this equation can be written in a table as follows:
| x | 5 | 4 | 3 |
| y | 1 | 0 | -1 |
The graphical representation is as follows:

From the graph, it can be observed that the two lines intersect each other at the point (7, 3).
So, x = 7 and y = 3.
Thus, the number of girls and boys in the class are 7 and 3 respectively.
(ii) Let the cost of one pencil and one pen be Rs x and Rs y respectively.
According to the given conditions, we have:
5x + 7y = 50
7x + 5y = 46
Three solutions of this equation can be written in a table as follows:
| x | 3 | 10 | -4 |
| y | 5 | 0 | 10 |
Three solutions of this equation can be written in a table as follows:
| x | 8 | 3 | -2 |
| y | -2 | 5 | 12 |
The graphical representation is as follows:

From the graph, it can be observed that the two lines intersect each other at the point (3, 5).
So, x = 3 and y = 5.
Therefore, the cost of one pencil and one pen are Rs 3 and Rs 5 respectively.
Concept insight: Read the question carefully and examine what are the unknowns. Represent the given conditions with the help of equations by taking the unknowns quantities as variables. Also carefully state the variables as whole solution is based on it. On the graph paper, mark the points accurately and neatly using a sharp pencil. Also, take at least three points satisfying the two equations in order to obtain the correct straight line of the equation. Since joining any two points gives a straight line and if one of the points is computed incorrect will give a wrong line and taking third point will give a correct line. The point where the two straight lines will intersect will give the values of the two variables, i.e., the solution of the two linear equations. State the solution point.
Chapter 3-Pairs of linear equation in 1 variable Exercise Ex.3.2
here it is solution of question 2
Solution 2
(i) 5x - 4y + 8 = 0
7x + 6y - 9 = 0
Comparing these equations with a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0, we get:
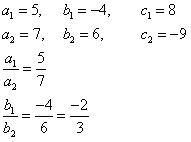
Since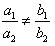 , the given pair of equations intersect at exactly one point.
, the given pair of equations intersect at exactly one point.
(ii) 9x + 3y + 12 = 0
18x + 6y + 24 = 0
Comparing these equations with a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0, we get:
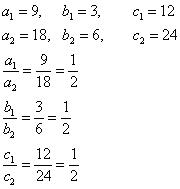
Since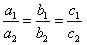 , the given pair of equation are coincident.
, the given pair of equation are coincident.
(iii) 6x - 3y + 10 = 0 , 2x - y + 9 = 0
Comparing these equations with a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0, we get:
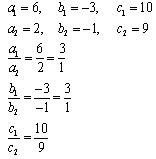
Since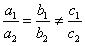 , the given pair of equation are parallel to each other.
, the given pair of equation are parallel to each other.
Concept insight: In order to answer such questions, remember the condition for the pair of linear equations to be intersecting, parallel or coincident. Also, while writing the coefficients, don't forget to take the signs.
7x + 6y - 9 = 0
Comparing these equations with a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0, we get:
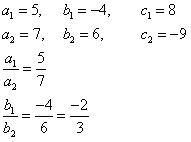
Since
(ii) 9x + 3y + 12 = 0
18x + 6y + 24 = 0
Comparing these equations with a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0, we get:
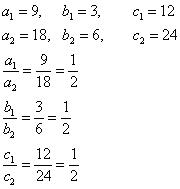
Since
(iii) 6x - 3y + 10 = 0 , 2x - y + 9 = 0
Comparing these equations with a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0, we get:
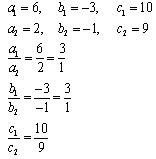
Since
Concept insight: In order to answer such questions, remember the condition for the pair of linear equations to be intersecting, parallel or coincident. Also, while writing the coefficients, don't forget to take the signs.
Chapter 3-Pairs of linear equation in 1 variable Exercise Ex.3.2
here it is solution of question 3
Solution 3
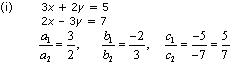
Since
Thus, the pair of linear equations is consistent.
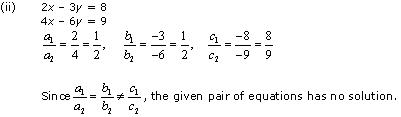
Thus, the pair of linear equations is inconsistent.
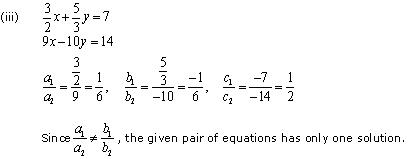
Thus, the pair of linear equations is consistent.
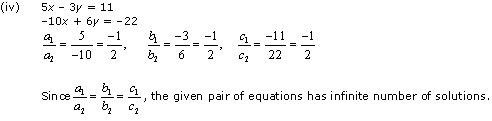
Thus, the pair of linear equations is consistent.
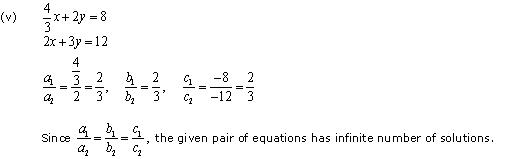
Thus, the pair of linear equations is consistent.
Concept Insight: If a pair of linear equations has one or more than one solution then they are said to be consistent and if they have no solution then they are said to be inconsistent. So, to identify the consistency of a given pair of equations, apply the conditions involving the coefficients of the given pair of equations.
In case, two consistent linear equations are plotted, they will either intersect or overlap each other.
Chapter 3-Pairs of linear equation in 1 variable Exercise Ex.3.2
here it is solution of question 4
Solution 4
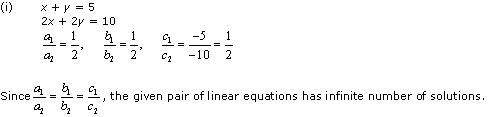
Thus, the pair of linear equations is consistent.
Now, x + y = 5
Three solutions of this equation can be written in a table as follows:
| x | 4 | 3 | 2 |
| y | 1 | 2 | 3 |
Three solutions of this equation can be written in a table as follows:
| x | 4 | 3 | 2 |
| y | 1 | 2 | 3 |
Thus, the graphical representation is as follows:

From the graph, it can be observed that the two lines coincide. Thus, the given pair of equations has infinite solutions.
Let x = k, then y = 5 - k. So, the ordered pair (k, 5 - k) , where k is a constant, will be the solution of the given pair of linear equations.
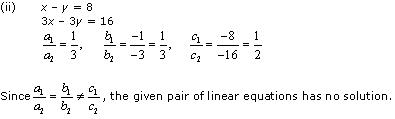
Thus, the pair of linear equations is inconsistent.
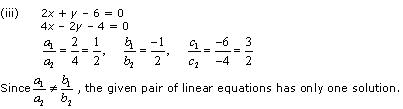
Thus, the pair of linear equations is consistent.
Now, 2x + y - 6 = 0
Three solutions of this equation can be written in a table as follows:
| x | 0 | 1 | 2 |
| y | 6 | 4 | 2 |
Three solutions of this equation can be written in a table as follows:
| x | 1 | 2 | 3 |
| y | 0 | 2 | 4 |
Thus, the graphical representation is as follows:

From the graph, it can be observed that the two lines intersect each other at the point (2, 2). Thus, the solution of the given pair of equations is (2, 2).
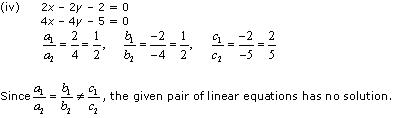
Thus, the pair of linear equations is inconsistent.
Concept insight: If a pair of linear equations has one or more than one solutions then they are said to be consistent and if they have no solution then they are said to be inconsistent. The graph of each equation can be plotted by taking at least three ordered pairs which are the solutions of the equations. The point where both the lines intersect will be the solution of the given pair of equations. Remember two overlapping lines intersect each other at infinitely many points. State the solution explicitly.
Chapter 3-Pairs of linear equation in 1 variable Exercise Ex.3.2
here it is solution of question 5
Solution 5
Let the width and length of the rectangular garden be x and y respectively.
According to the given conditions,
y - x = 4
y + x = 36
y - x = 4 y = x + 4
y = x + 4
Three solutions of this equation can be written in a table as follows:
y + x = 36
Three solutions of this equation can be written in a table as follows:
Thus, the graphical representation is as follows:
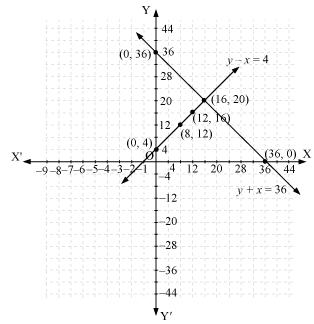
From the graph, it can be observed that the two lines intersect each other at the point (16, 20). So, x = 16 and y = 20.
Thus, the length and width of the rectangular garden is 20 m and 16 m respectively.
Concept insight: Here dimensions of the rectangular garden needs to be found. Since opposite sides of the rectangle are equal so length and breadth can be taken as variables. Applying conditions given in the problem two linear equations in the 2 variables can be obtained. Now, in order to represent the obtained equations graphically, take the values of variables as whole numbers only, because then it will be easier to represent the values on the graph. The point where the two equations intersect will give the required dimensions. State the dimensions length and breadth from the values of the variables.
According to the given conditions,
y - x = 4
y + x = 36
y - x = 4
Three solutions of this equation can be written in a table as follows:
| x | 0 | 8 | 12 |
| y | 4 | 12 | 16 |
y + x = 36
Three solutions of this equation can be written in a table as follows:
| x | 0 | 36 | 16 |
| y | 36 | 0 | 20 |
Thus, the graphical representation is as follows:

From the graph, it can be observed that the two lines intersect each other at the point (16, 20). So, x = 16 and y = 20.
Thus, the length and width of the rectangular garden is 20 m and 16 m respectively.
Concept insight: Here dimensions of the rectangular garden needs to be found. Since opposite sides of the rectangle are equal so length and breadth can be taken as variables. Applying conditions given in the problem two linear equations in the 2 variables can be obtained. Now, in order to represent the obtained equations graphically, take the values of variables as whole numbers only, because then it will be easier to represent the values on the graph. The point where the two equations intersect will give the required dimensions. State the dimensions length and breadth from the values of the variables.
Chapter 3-Pairs of linear equation in 1 variable Exercise Ex.3.2
here it is solution of question 6
Solution 6
(i) For the two lines a1x + b1x + c1 = 0 and a2x + b2x + c2 = 0, to be intersecting, we must have
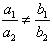
So, the other linear equation can be 5x + 6y - 16 = 0
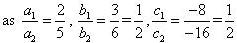
(ii) For the two lines a1x + b1x + c1 = 0 and a2x + b2x + c2 = 0, to be parallel, we must have
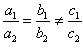
So, the other linear equation can be 6x + 9y + 24 = 0,
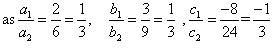
(iii) For the two lines a1x + b1x + c1 = 0 and a2x + b2x + c2 = 0 to be coincident, we must have
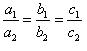
So, the other linear equation can be 8x + 12y - 32 = 0,
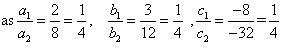
Concept insight: In order to answer such type of problems, just remember the conditions for two lines to be intersecting, parallel, and coincident. This problem will have multiple answers as their can be many equations satisfying the required conditions.
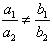
So, the other linear equation can be 5x + 6y - 16 = 0
(ii) For the two lines a1x + b1x + c1 = 0 and a2x + b2x + c2 = 0, to be parallel, we must have
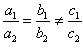
So, the other linear equation can be 6x + 9y + 24 = 0,
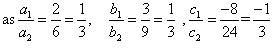
(iii) For the two lines a1x + b1x + c1 = 0 and a2x + b2x + c2 = 0 to be coincident, we must have
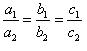
So, the other linear equation can be 8x + 12y - 32 = 0,
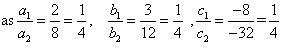
Concept insight: In order to answer such type of problems, just remember the conditions for two lines to be intersecting, parallel, and coincident. This problem will have multiple answers as their can be many equations satisfying the required conditions.
Chapter 3-Pairs of linear equation in 1 variable Exercise Ex.3.2
here it is solution of question 7
Solution 7
x - y + 1 = 0 Three solutions of this equation can be written in a table as follows:
| x | 0 | 1 | 2 |
| y | 1 | 2 | 3 |
Three solutions of this equation can be written in a table as follows:
| x | 4 | 2 | 0 |
| y | 0 | 3 | 6 |
Now, these equations can be drawn on a graph. The triangle formed by the two lines and the x-axis can be shown by the shaded part as:

From the graph, it can be observed that the coordinates of the vertices of the triangle so formed are (2, 3), (-1, 0), and (4, 0).
Concept insight: In order to find the coordinates of the vertices of the triangle so formed, find the points where the two lines intersects the x-axis and also where the two lines intersect each other. Note here that the coordinates of the intersection of lines with x-axis is taken and not with y-axis, this is because the question says to find the triangle formed by the two lines and the x-axis.
Dont Forget to subscribe my channel.
this are the link of previous video-
Part 1 Electricity Class 10-
https://youtu.be/JvGpUNF5dhA
Class 10 physics s chand-
https://amzn.to/2ygIG5a
Interesting question video-
https://mydomainscan.com/ZsbiLmG
Maths problem-
https://mydomainscan.com/wxSr5VQg
motivation-
https://mydomainscan.com/wxSr5VQg
best books-
https://amzn.to/3fThv1f
https://amzn.to/2LEbPKA
if you not read the previous blog please read it. it is the solution to exercise 1.1 AND EXERCISE 2.3
not forget to suscribe our channel. also follow us on the telegram grouup
Here at Subject Wise Our Focus Is To Provide Best Quality Education To All Students From India And Across The Globe.
------------------------------------------------------------------------------------------------------------
Follow Us On Our Social Media Handles For Behind The Scenes Stuff And Important Updates....
TELEGRAM 👇🏼
https://t.me/joinchat/K40m2h2t4kDvXN1ATNYUqg
Blogger 👇🏼
https://subjectwiseyt.blogspot.com/
-------------------------------------------------------------------------------------------------------------
If This Video Was Helpful Do Leave A Like Since It Encourages Us To Make More Videos For All You Wonderful Students.
Make Sure To Comment Your Views Below.
Lastly Make Sure To Subscribe Our Channel And Press The Bell Icon For All Notification Of Our Latest Videos.
-------------------------------------------------------------------------------------------------------------
For Live Sessions, Chapter wise notes and Important Question Bank make sure you register at
https://subjectwiseyt.blogspot.com/
Get Your Courses On Our Website : For A Distraction Free And Efficient Studies
-------------------------------------------------------------------------------------------------------------
Please help me and tell me all the mistakes committed by me so i can improve it in next video.
Dont Forget to subscribe my channel.
this are the link of previous video-
Basics of Biology Part 1-
https://www.youtube.com/watch?v=R3UzzSq-h2Y
Part2- Electricity Class 10-
https://www.youtube.com/watch?v=Wjsw6GuJnz0&list=PLcMqfTNwNJuQ6ut9yqZwWaHiaPRgJIJ57&index=2
Part 1 Electricity Class 10-
https://youtu.be/JvGpUNF5dhA
Class 10 physics s chand-
https://amzn.to/2ygIG5a
Interesting question video-https://mydomainscan.com/ZsbiLmG
Maths problem-
https://mydomainscan.com/wxSr5VQg
motivation-
https://mydomainscan.com/wxSr5VQg
best books-
https://amzn.to/3fThv1f
https://amzn.to/2LEbPKA
Next video will definetly come within one week
------------------------------------------------------------------------------------------------
SubjectWise - New way to learn
------------------------------------------------------------------------------------------------
At last Thanks for watching Our Videos For any new Requirement please Comment Below.
#Class10Crack #FightagainstCorona #SubjectWise

Comments
Post a Comment